y ' - t^2 y = 0
****
#$&*
y ' - y = 0
****
#$&*
y' - y / t = 0
****
#$&*
y ' - t y = 0
****
#$&*
y ' + t y = 0
****
#$&*
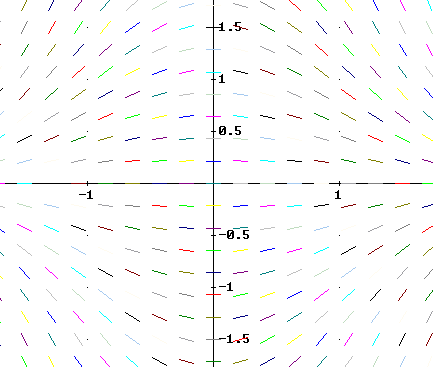
A
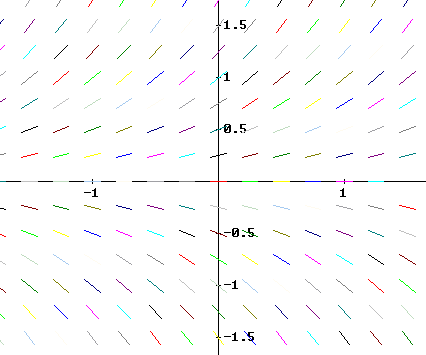
B

C

D
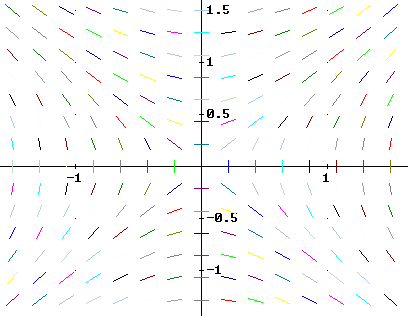
E

F
Section 2.2
Question: 1. Solve the following equations with the given initial conditions:
1. y ' - 2 y = 0, y(1) - 3
Your solution:
Confidence rating:
Given Solution:
Self-critique (if necessary):
Self-critique rating:
Question: 2. t^2 y ' - 9 y = 0, y(1) = 2.
Your solution:
Confidence rating:
Given Solution:
Self-critique (if necessary):
Self-critique rating:
Question: 3. (t^2 + t) y' + (2t + 1) y = 0, y(0) = 1.
Your solution:
Confidence rating:
Given Solution:
Self-critique (if necessary):
Self-critique rating:
Question: 4. y ' + sin(3 t) y = 0, y(0) = 2.
Your solution:
Confidence rating:
Given Solution:
Self-critique (if necessary):
Self-critique rating:
Question: 5. Match each equation with one of the direction fields shown below, and explain why you chose as you did.
y ' - t^2 y = 0
****
#$&*
y ' - y = 0
****
#$&*
y' - y / t = 0
****
#$&*
y ' - t y = 0
****
#$&*
y ' + t y = 0
****
#$&*
A
B
C
D
E
F
6. The graph of y ' + b y = 0 passes through the points (1, 2) and (3, 8). What is the value of b?
Your solution:
Confidence rating:
Given Solution:
Self-critique (if necessary):
Self-critique rating:
Question:
7. The equation y ' - y = 2 is first-order linear, but is not homogeneous.
If we let w(t) = y(t) + 2, then:
What is w ' ?
****
#$&*
What is y(t) in terms of w(t)?
****
#$&*
What therefore is the equation y ' - y = 2, written in terms of the function w and its derivative w ' ?
****
#$&*
Now solve the equation and check your solution:
Solve this new equation in terms of w.
****
#$&*
Substitute y + 2 for w and get the solution in terms of y.
****
#$&*
Check to be sure this function is indeed a solution to the equation.
****
#$&*
Your solution:
Confidence rating:
Given Solution:
Self-critique (if necessary):
Self-critique rating:
Question: 8. The graph below is a solution of the equation y ' - b y = 0 with initial condition y(0) = y_0. What are the values of y_0 and b?
Your solution:
Confidence rating:
Given Solution:
Self-critique (if necessary):
Self-critique rating: