If your solution to stated problem does not match the given solution, you should self-critique per instructions at
http://vhcc2.vhcc.edu/dsmith/geninfo/labrynth_created_fall_05/levl1_22/levl2_81/file3_259.htm
.
Your solution, attempt at solution. If you are unable to attempt a solution, give a phrase-by-phrase interpretation of the problem along with a statement of what you do or do not understand about it. This response should be given, based on the work you did in completing the assignment, before you look at the given solution.
007. Triangular, Square, Pentagonal Numbers
Question: `q001. Note that there are 10 questions in this assignment.
Sketch three points A, B and C forming an equilateral triangle on a piece of paper, with point A at the lower left-hand corner, point B at the lower right-hand corner and point C at the top. Sketch the segments AB and AC.
Now double the lengths of AB and AC, and place a point at each of the endpoints of these segments. Connect these new endpoints to form a new equilateral triangle. Two sides of this triangle will have three points marked while the new side will only have its two endpoints marked. Fix that by marking that middle point, so all three sides of your new triangle are marked the same.
How many marked points were there in the original triangle, and how many are there in the new triangle?
Your solution:
Confidence Assessment:
Given Solution:
The original triangle had the three points A, B and C. When you extended the two sides you marked the new endpoints, then you marked the point in the middle of the third side. So you've got 6 points marked.
The construction of these numbers is shown in the figure below. We begin with a single dot:
![]()
We label this point A and construct a triangle containing this point as a vertex. We place similar dots at the vertices of this triangle.

We now 'scale up' the triangle by doubling the lengths of its sides:
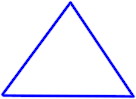
We divide this triangle into triangles of the original size, and place dots at each of these vertices.
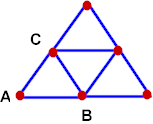
The first figure has a single 'dot', the second has 3 'dots', and the third has 6 'dots'.
Note the similarity with the figures below.
The first depicts the pattern illustrated in this question.

The second illustrates the pattern extended one steps:

The third depicts the pattern as it would appear if extended 12 steps beyond the original triangle:

Self-critique (if necessary):
Self-critique Rating:
Question: `q002. Extend the two sides that meet at A by distances equal to the original lengths AC and AB and mark the endpoints of the newly extended segments. Each of the newly extended sides will have 4 marked points. Now connect the new endpoints to form a new right triangle. Mark points along the new side at the same intervals that occur on the other two sides. How many marked points are on your new triangle, and how many in the whole figure?
Your solution:
Confidence Assessment:
Given Solution:
You added the two new endpoints when you extended the sides. You then should have marked two new points on the new third side, so that each side contains 4 points including its endpoints. Your figure will now contain 10 marked points.
The construction is shown below. First we extend the two sides by a length equal to that of the original triangle:
Next we join the 'free' endpoints of those new sides to form a triangle.
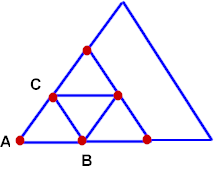
Now we place points along the new side and join them to complete the 'small' triangles within our new figure:
We have added four new dots.
The figure below depicts only the 'dots', without the triangles:

Self-critique (if necessary):
Self-critique Rating:
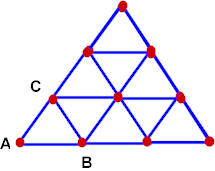
Question: `q003. Continue the process for another step-extend each side by a distance equal to the original point-to-point distance. How many points do you have in the new triangle?
Your solution:
Confidence Assessment:
Given Solution:
You will add an endpoint to each newly extended side, so each of the new sides will contain 5 points. You will then have to add 3 equally spaced points to the new side, giving you a total of 13 points on the new triangle. In addition there are two marked points inside the triangle, for a total of 15 points.
Click on 'Next Picture' to see the construction. The line segments along two sides of the triangle have again been extended and points marked at the ends of these segments. The new endpoints have been connected to form the third side of a larger triangle, and equally spaced points have been constructed along that side.
`routine triangle4
Self-critique (if necessary):
Self-critique Rating:
Question: `q004. Continue the process for one more step. How many points do you have in the new triangle?
Your solution:
Confidence Assessment:
Given Solution:
You will add an endpoint to each newly extended side, so each of the new sides will contain 6 points. You will then have to add 4 equally spaced points to the new side, giving you a total of 15 points on the new triangle. There are also 5 marked points inside the triangle for a total of 21 marked points.
Self-critique (if necessary):
Self-critique Rating:
Question: `q005. The sequence of marked points is 3, 6, 10, 15, 21. What do expect will be the next number in this sequence?
Your solution:
Confidence Assessment:
Given Solution:
The differences between these numbers are 3, 4, 5, 6. The next difference, according to this pattern, should be 7, which would make the next number 28.
Self-critique (if necessary):
Self-critique Rating:
Question: `q006. How can you tell, in terms of the process you used to construct these triangles, that the next number should be 7 greater?
Your solution:
Confidence Assessment:
Given Solution:
When you extend the triangle again, you will add two new endpoints and each side will now have 7 points. The 7 points on the new triangle will be all of the new points.
Self-critique (if necessary):
Self-critique Rating:
Question: `q007. How do you know this sequence will continue in this manner?
Your solution:
Confidence Assessment:
Given Solution:
Each time you extend the triangle, each side increases by 1. All the new marked points are on the new side, so the total number of marked points will increase by 1 more than with the previous extension.
Self-critique (if necessary):
Self-critique Rating:
The picture below depicts this sequence extended to the 24th number.
You should understand why the number of beads in this picture is 1 + 2 + 3 + 4 + 5 + ... + 21 + 22 + 23 + 24.

Question: `q008. How many BB's would there be if the top half of the triangle in the above picture was removed?
Your solution:
Confidence Rating:
The last two questions are a little more challenging than most q_a_ questions. See if you can get them.
Question: `q009. A triangle similar to the above triangle, but containing about twice as many rows, would contain 725 BB's. If another row is added there will be 776 BB's. How many rows are there in each of the triangles?
Your solution:
Confidence Rating:
Question: `q010. The formula
T_n = 1/2 * n * (n+1)
can be used to find the number of BB's in a triangle with n rows (this number is called the nth triangular number).
So for example the fourth triangular number is
T_4 = 1/2 * 4 * (4 + 1) = 10.
The sequence 1, 3, 6, 10, 15, 21, ... of triangular numbers could be written in symbols as T_1, T_2, T_3, T_4, T_5, T_6, ... .
Use the formula to find the 14th and the 15th triangular numbers, and calculate the difference between them. Why did you get the difference you did?
Your solution:
Confidence Rating:
Self-critique Rating: