If your solution to stated problem does not match the given solution, you should self-critique per instructions at
http://vhcc2.vhcc.edu/dsmith/geninfo/labrynth_created_fall_05/levl1_22/levl2_81/file3_259.htm
.
Your solution, attempt at solution.† If you are unable to attempt a solution, give a phrase-by-phrase interpretation of the problem along with a statement of what you do or do not understand about it.† This response should be given, based on the work you did in completing the assignment, before you look at the given solution.
004. Extending to circle of radius 3
Goals for this Assignment include but are not limited to the following:
1. Construct a table of the values of y = A sin(x) for a given value of A, extending for a complete cycle of this function, with x equal to multiples of pi/6 or pi/4, and using the table construct a graph of one cycle of y = A sin(x ).
2. Given a function y = A sin(theta) with theta given as a function of x, construct a table of the values of y = A sin(theta) for a complete cycle of this function with theta equal to multiples of pi/6 or pi/4, then determine the x value corresponding to each value of theta. Using a table of y vs. x construct a graph of one cycle of y = A sin(theta) in terms of the given function theta of x, clearly labeling the x axis for each quarter-cycle of the function.
3. Interpret the function and graph corresponding to Goal 2 in terms of angular motion on a unit circle.
Click once more on Next Question/Answer for a note on Previous Assignments.
Previous Assignments: Be sure you have completed Assignment 3 as instructed under the Assts link on the homepageand submitted the result of the Query and q_a_ from that Assignment.
The questions in this assignment continue those in the preceding assignment. For convenience you can locate the preceding assignment by clicking on link to preceding assignment.
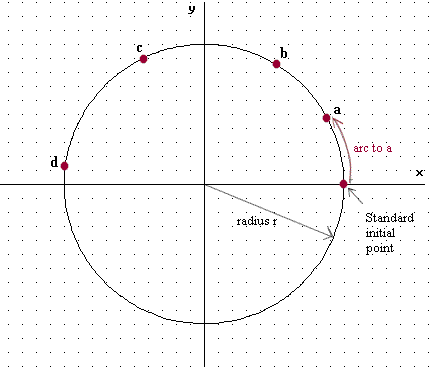
Question: `q001. In the preceding assignment we saw how to model the sine function using a circle of radius 1.
Now we consider a circle of radius 3.
An angular position of 1 radian again corresponds to an arc displacement equal to the radius of the circle.
Which point on the circle in the picture corresponds to the angular position of 1 radian?

Your solution:
Confidence Assessment:
Given Solution:
The distance along the arc will be equal to the radius at point b. So the angular position of one radian occurs at point b.
We see that when the circle is scaled up by a factor of 3, the radius becomes 3 times as great so that the necessary displacement along the arc becomes 3 times as great.
Note that the 1-radian angle therefore makes the same angle as for a circle of radius 1. The radius of the circle doesn't affect the picture; the radius simply determines the scale at which the picture is interpreted.
Self-critique (if necessary):
Self-critique Rating:
Question: `q002. On the circle of radius 3 what arc distance will correspond to an angle of pi/6?
Your solution:
Confidence Assessment:
Given Solution:
`aOn a circle of radius 1 the arc distance pi/6 corresponds to an arc displacement of pi/6 units. When the circle is scaled up to radius 3 the arc distance will become three times as great, scaling up to 3 * pi/6 = pi/2 units.
Self-critique (if necessary):
Self-critique Rating:
Question: `q003. If the red ant is moving along a circle of radius 3 at a speed of 2 units per second, then what is its angular velocity--i.e., its the rate in radians / second at which its angular position changes?
Your solution:
Confidence Assessment:
Given Solution:
`aSince 3 units corresponds to one radian, 2 units corresponds to 2/3 radian, and 2 units per second will correspond to 2/3 radian/second.
STUDENT QUESTION
I have this in my notes and Iíve reviewed the text section
and I donít understand why this is. I may be making this more difficult than it
is.
INSTRUCTOR RESPONSE
@&
A distance on the arc which is equal to the radius corresponds to a radian of
angle.
So for this circle it takes 3 units of distance on the arc to correspond to one
radian.
The ant moves 2 units of distance on the arc every second. That corresponds to
less than a radian, since a radian corresponds to 3 units of distance.
So the ant is moving at less than a radian per second.
It is in fact moving at 2/3 of a radian per second.
Be sure to let me know if this is still unclear.
*@
Self-critique (if necessary):
Self-critique Rating:
Question: `q004. If the red ant is moving along at angular velocity 5 radians/second on a circle of radius 3, what is its speed?
Your solution:
Confidence Assessment:
Given Solution:
`aEach radian on a circle of radius 3 corresponds to 3 units of distance. Therefore 5 radians corresponds to 5 * 3 = 15 units of distance and 5 radians/second corresponds to a speed of 15 units per second.
Self-critique (if necessary):
Self-critique Rating:
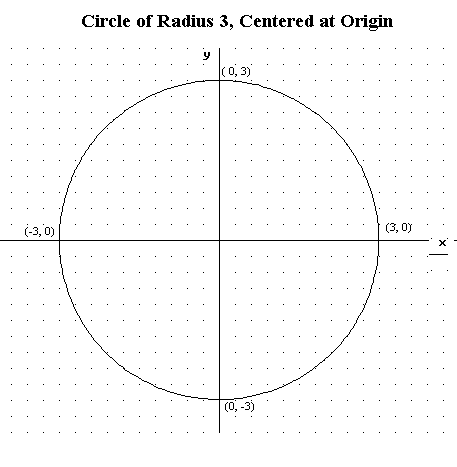
Question: `q005. Figure 17 shows a circle of radius 3 superimposed on a grid with .3 unit between gridmarks in both x and y directions. Verify that this grid does indeed correspond to a circle of radius 3.
Estimate the y coordinate of each of the points whose angular positions correspond to 0, pi/6, pi/3, pi/2, 2 pi/3, 5 pi/6, pi, 7 pi/6, 4 pi/3, 3 pi/2, 5 pi/3, 11 pi/6 and 2 pi.
Your solution:
Confidence Assessment:
Given Solution:
The angular positions of the points coinciding with the positive and negative x axes all have y coordinate 0; these angles include 0, pi and 2 pi. At angular position pi/2 the y coordinate is equal to the radius 3 of the circle; at 3 pi/2 the y coordinate is -3. At angular position pi/6 the point on the circle appears to be close to (2.7,1.5); the x coordinate is actually a bit less than 2.7, perhaps 2.6, so perhaps the coordinates of the point are (2.6, 1.5). Any estimate close to these would be reasonable.
The y coordinate of the pi/6 point is therefore 1.5.
The coordinates of the pi/3 point are (1.5, .87), just the reverse of those of the pi/6 point; so the y coordinate of the pi/3 point is approximately 2.6.
The 2 pi/3 point will also have y coordinate approximately 2.6, while the 4 pi/3 and 5 pi/3 points will have y coordinates approximately -2.6. The 5 pi/6 point will have y coordinate 1.5, while the 7 pi/6 and 11 pi/6 points will have y coordinate -1.5.
Self-critique (if necessary):
Self-critique Rating:
Question: `q006. The y coordinates of the unit-circle positions 0, pi/6, pi/3, pi/2, 2 pi/3, 5 pi/6, pi, 7 pi/6, 4 pi/3, 3 pi/2, 5 pi/3, 11 pi/6 and 2 pi are 0, .5, .87, 1, .87, .5, 0, -.5, -.87, -1, -.87, -.5, 0. What should be the corresponding y coordinates of the points lying at these angular positions on the circle of radius 3? Are these coordinates consistent with those you obtained in the preceding problem?
Your solution:
Confidence Assessment:
Given Solution:
`aOn a radius 3 circle the y coordinates would each be 3 times as great. The coordinates would therefore be obtained by multiplying the values 0, .5, .87, 1, .87, .5, 0, -.5, -.87, -1, -.87, -.5, 0 each by 3, obtaining 0, 1.5, 2.61, 3, 2.61, 1.5, 0, -1.5, -2.61, -3, -2.61, -1.5, 0.
These values should be close, within .1 or so, of the estimates you made for this circle in the preceding problem.
Self-critique (if necessary):
Self-critique Rating:
Question: `q007. The exact y coordinates of the unit-circle positions 0, pi/6, pi/3, pi/2, 2 pi/3, 5 pi/6, pi, 7 pi/6, 4 pi/3, 3 pi/2, 5 pi/3, 11 pi/6 and 2 pi are 0, .5, sqrt(3) / 2, 1, sqrt(3) / 2, .5, 0, -.5, -sqrt(3) / 2, -1, -sqrt(3) / 2, -.5, 0.
What should be the corresponding y coordinates of the points lying at these angular positions on the circle of radius 3?
Are these coordinates consistent with those you obtained in the preceding problem?
Your solution:
Confidence Assessment:
Given Solution:
On a radius 3 circle the y coordinates would each be 3 times as great. The coordinates would therefore be obtained by multiplying the values
0, .5, sqrt(3) / 2, 1, sqrt(3) / 2, .5, 0, -.5, -sqrt(3) / 2, -1, -sqrt(3) / 2, -.5, 0
each by 3, obtaining
0, 1.5, 3 sqrt(3) / 2, 3, 3 sqrt(3) / 2, 1.5, 0, -3 sqrt(3) / 2, -3 sqrt(3) / 2, -3, -2.61, -1.5, 0.
Self-critique (if necessary):
Self-critique Rating:
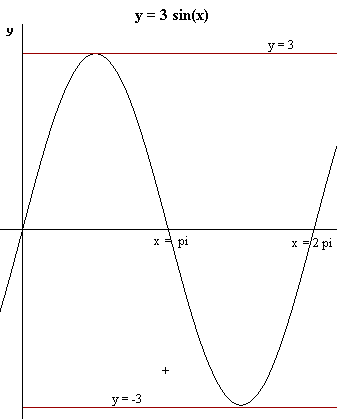
Question: `q008. Sketch a graph of the y coordinate obtained for a circle of radius 3 in the preceding problem vs. the anglular position theta.
Your solution:
Confidence Assessment:
Given Solution:
`aYour graph should be as shown in Figure 54. This graph as the same description as a graph of y = sin(theta) vs. theta, except that the slopes are all 3 times as great and the maximum and minimum values are 3 and -3, instead of 1 and -1.
Self-critique (if necessary):
Self-critique Rating:
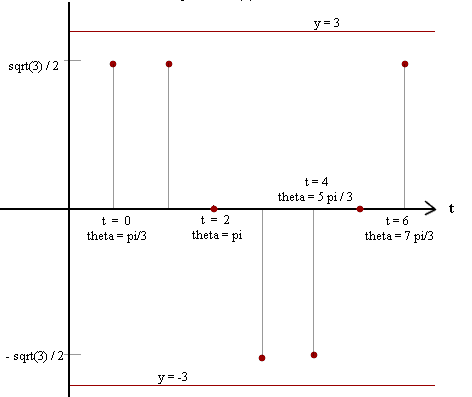
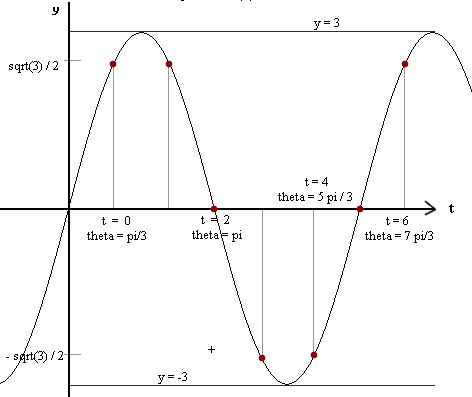
Question: `q009. If the red ant starts on the circle of radius 3, at position pi/3 radians, and proceeds at pi/3 radians per second then what will be its angular position after 1, 2, 3, 4, 5 and 6 seconds? What will be the y coordinates at these points? Make a table and sketch a graph of the y coordinate vs. the time t. Describe the graph of y position vs. clock time.
Your solution:
Confidence Assessment:
Given Solution:
`aThe angular positions at t = 1, 2, 3, 4, 5 and 6 are 2 pi/3, pi, 4 pi/3, 5 pi/3, 2 pi and 7 pi/3. The corresponding y coordinates are 3 * sqrt(3) / 2, 0, -3 * sqrt(3) / 2, -3 * sqrt(3) / 2, 0 and 3 * sqrt(3) / 2.
If you just graph the corresponding points you will miss the fact that the graph also passes through y coordinates 3 and -3; from what you have seen about these functions in should be clear why this happens, and it should be clear that to make the graph accurate you must show this behavior. See these points plotted in red in Figure 45, with the t = 0, 2, 4, 6 values of theta indicated on the graph.
The graph therefore runs through its complete cycle between t = 0 and t = 6, starting at the point (0, 3 * sqrt(3) / 2), or approximately (0, 2.6), reaching its peak value of 3 between this point and (1, 3 * sqrt(3) / 2), or approximately (1, 2.6), then reaching the x axis at t = 3 as indicated by the point (2, 0) before descending to (3, -3 * sqrt(3) / 2) or approximately (3, -2.6), then through a low point where y = -3 before again rising to (4, -3 * sqrt(3) / 2) then to (5, 0) and completing its cycle at (6, 3 * sqrt(3) / 2). This graph is shown in Figure 86.
Self-critique (if necessary):
Self-critique Rating:
Question: `q010. In this question and the next we will construct a table and a y vs. t graph for the function y = 3 sin(pi/4 * t + pi/3).
We first construct a table for y = 3 sin(theta) vs. theta. Make a table for the graph of y = 3 sin(theta), using theta = 0, pi/4, pi/2, etc., and plot the corresponding graph. However, when making your table, the first column should be for the variable t; for the purposes of the present question this first column will be blank, except for the heading t (this column will be filled in during the next step).
Your table will look something like the one below, with values for theta, sin(theta) and 3 sin(theta) filled in, and the column for t blank.
| t | theta | sin(theta) | 3 sin(theta) |
Describe your graph of 3 sin(theta) vs. theta.
Note that you have constructed a table and graph of y vs. theta, not y vs. t. The variable t is different than the variable theta, as should be clear from your table.
Now we find the values of t necessary to achieve the given values of theta.
Your table shows values of 3 sin(theta) vs. theta.
We want a table for 3 sin(pi/4 * t + pi/3) vs. t.
We can accomplish this by simply letting theta = pi/4 * t + pi/3.
Solve this equation for t.
Then plug each of your chosen theta values into your solution, and use these values to fill in the t column of your table.
If you relabel the horizontal axis of your graph with the corresponding t values, you will have a graph of the function y = 3 sin(pi/4 * t + pi/3).
Your solution:
Confidence Assessment:
Given Solution:
`aUsing columns for t, theta and sin(theta) as we have done before, and an additional column for 3 * sin(theta) we obtain the following initial table:
t theta = pi/4 t + pi/3 sin(theta) 3 * sin(theta)
0 0
pi/4 .71
pi/2 0
3 pi/4 .71
pi 0
5 pi/4 -.71
3 pi/2 -1
7 pi/4 -.71
2 pi 0 0
The solution to
pi/4 t + pi/3 = theta is obtained by first adding -pi/3 to both sides to obtain
pi/4 t = theta - pi/3, then multiplying both sides by 4 / pi to obtain
t = 4 / pi * theta - 4 / pi * pi/3, and finally simplifying to get
t = 4 / pi * theta - 4/3.
Substituting in the given values of theta we obtain t values -4/3, -1/3, 2/3, 5/3, 8/3, 11/3, 14/3, 17/3, 20/3.
We also multiply the values of sin(theta) by 3 to get the values of 3 sin(theta):
t theta = pi/4 t + pi/3 sin(theta) 3 * sin(theta)
-4/3 0 0 0
-1/3 pi/4 .71 2.1
2/3 pi/2 0 0
5/3 3 pi/4 .71 2.1
8/3 pi 0 0
11/3 5 pi/4 -.71 -2.1
14/3 3 pi/2 -1 -1
17/3 7 pi/4 -.71 -2.1
20/3 2 pi 0 0
Since theta = pi/4 t + pi/3, if we graph the final column vs. the first we have the graph of y = 3 sin(pi/4 t + pi/3) vs. t. This graph is shown in Figure 19, with red dots indicating points corresponding to rows of the table.
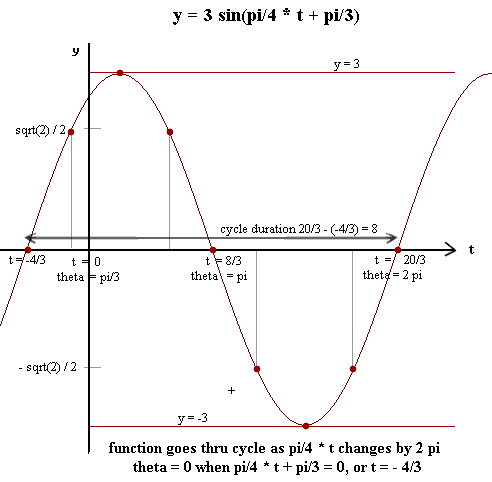
The graph is as in the figure (fig 3 sin(pi/4 * t + pi/3)), with a standard cycle running from t = -4/3 to t = 20/3. During this cycle y goes from 0 to 3 to 0 to -3 to 0. The duration of the cycle is 20/3 - (-4/3) = 24/3.
Complete Assignment 4, including Class Notes, text problems and Web-based problems as specified on the Assts page.
When you have completed the entire assignment run the Query program. Submit SEND files from Query and q_a_.