In a certain city, the length of the daylight runs from 9.6 hours to 14.4 hours on an annual cycle.

• The circular model for y = day length is therefore y = A sin( `omega (t - h) ) + C, with A = 2.4 and C = 12.
• Since the period of the day-length cycle is 365 days, `omega = 2 `pi / 365.
• The day-length cycle is shifted to the right by 80 days so that we have an increasing 12-hour day length on the 80th day of the year, so h = 80.
• The result is the model given the figure below.

We wish to determine when the day length is 10 hours.

• We set y = day length = 10 and solve the resulting equation.
• After doing some simple algebra we arrive at sin( 2 `pi / 365 (t - 80 ) ) = -.833....
• We write down the solutions using the inverse sine function, as indicated in the last line.

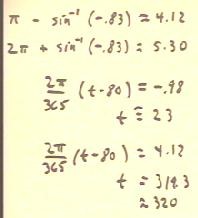
The figure below depicts the circular model and the graph of y vs. t, showing the positions on the reference circle and on the graph where y = day length = 10.

• The reference circle, of radius 2.4, is centered at y = 12.
• The graph is shifted 80 units to the right, with its midpoint y value occurring at t = 80, where y is increasing.

The graph below depicts fullness vs. clock time t for 1 cycle of the moon, with time duration 28 days and hence `omega = 2 `pi / 28.

• The fullness y of the moon goes from 0% to 100%, so the reference circle is centered at y = 50 and has radius 50.
• If we wish to determine for how long the moon is at least 99% full.
• We therefore set y = 99 and solve the resulting equation, as indicated.
• Our solution indicates that y = 99 at t = 6.1 days and at t = 7.9 days during the cycle, so that the moon remains at least 99% full for 1.8 days.

An observer 20 feet from an actor on the stage wishes to determine how much of her field of vision is filled by the glorious sight.

• The actor, as indicated, is 68 inches tall and the eye level of the observer is 12 inches below the feet of the actor.
• The angles of the observer's line of sight to the feet and to the head of the actor, measured with respect to the horizontal direction, are the angles `theta and `alpha indicated in the figure below.
• `theta is the angle that determines how much of the observer's field of vision is occupied by the actor.

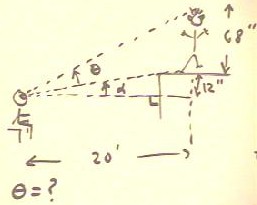
The right triangle below shows that the total angle `theta + `alpha between horizontal and the observer's line of sight to the top of the actor's head is tan^-1 (80 / 240) = 18.4 deg, while the angle between horizontal and the line of sight to the actor's feet is `alpha = tan^-1(12 / 240) = 2.9 deg.

• The desired angle `theta is therefore easily found by subtracting `alpha from `theta + `alpha.