Today we see

Bernoulli's equation is stated at the top of the figure below.

We note that the terms 1/2 `rho v^2 and `rho g h are reminiscent of the expressions for kinetic and gravitational potential energy.

During the last class we determined that when water flows from a hole in the side of a container, when water depth relative to the hole is h, then if no energy is dissipated the exit velocity of the water is found from energy conservation to be vExit = `sqrt(2 g h).

We use Bernoulli's equation to obtain the same result:

We define state 1 to be the velocity, altitude and pressure at the slowly descending water surface in the container.

• We say that P1 = 1 atm, h1 = 17 cm and v1 = 0.

We define state 2 to be the velocity, altitude and pressure of the water as it exits the container.

• At this point

• We say that P2 = 1 atm, h2 = 0 and v2 =vExit.

Bernoulli's equation says that 1/2 `rho v^2 + `rho g h + P is constant, which means that the value of this expression is the same for both states.

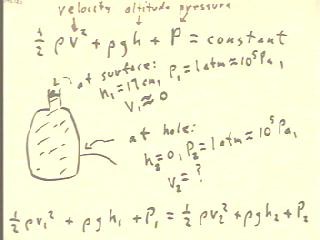
Since the pressures are equal, as indicated in the second line of the figure below, the pressure terms can be subtracted from both sides and thereby eliminated.

• The result is the equation in the third line, which relates velocity and altitude for the two states we have defined.

We rearrange the equation so that the kinetic energy terms are on one side and the potential energy terms on the other.

• In this rearrangement we see (second line from bottom of figure) that the change in the kinetic energy term is equal to the negative of the change in the potential energy term, which is a direct analogy to energy conservation in the absence of energy dissipation.
• We see also that when pressure is constant the sum of the kinetic and potential energy terms is constant, as indicated in the line at the bottom of the figure below.

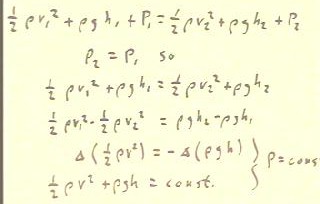
It follows that a decrease in the potential energy term is associated with an increase in the kinetic energy term.

• We again note that if we multiply by the volume V of the fluid element, we obtain its kinetic and potential energies so that the expression given that the bottom of the figure below expresses energy conservation.

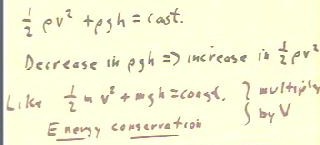
We can now solve for the exit velocity v2 the equation relating the velocities and altitudes for the two states we have defined:

• We begin with the equation in the first line and divide both sides by `rho.
• When we factor the 1/2 from the left-hand side and the g from the right-hand side we obtain the equation in the second line.
• Using the fact that the velocity v1 of the fluid in the first state is nearly 0, we obtain the equation in the third line.
• This equation is easily solved for v2, obtaining the expression in the second line from the bottom.
• We finally note that h2 = 0, so that the exit velocity is v2 = `sqrt( 2 g h1).
• We note that this is identical to the expression `sqrt(2 g h) obtained by energy considerations in the preceding class for the exit velocity, where h was the altitude of the fluid relative to the hole and is easily identified with the h1 of the present model.

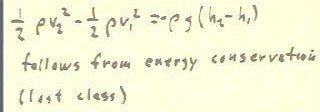
We now relate pressure P to the potential energy term `rho g h. 

If we consider a fluid filling a 'rectangular' container(called a 'rectangular parallelopiped'), we see that the bottom of the container must support the weight of all the fluid above it.

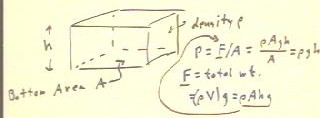
Bernoulli's Equation gives us the same result.

• We implicitly have assumed that the fluid is stationary in the container.
• It follows that only the P and `rho g h terms are changing, so that an increase in P is associated with a decrease in `rho g h.
• This is consistent with our experience of fluids, in which decreasing altitude (i.e., increasing depth) is associated with increasing pressure.
• The various forms of Bernoulli's equation for constant velocity are given that the bottom of the figure below.

Another application of Bernoulli's Equation is depicted below, where we see that increasing velocity can be associated with decreasing pressure.

• If water is moving through a closed pipe which narrows at a certain point, then assuming that the water fills the pipe, it should be clear that to carry the same amount of flow its velocity must be greater in the narrower part of the pipe.
• If the pipe is horizontal, then there is no significant difference in the altitude h of the water from the wider to the narrower section.
• It follows that the resulting increase in the kinetic energy term 1/2 `rho v^2 must be associated with a decrease in the pressure P.

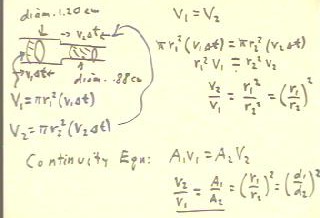
We quantify the nature of the velocity change:

• Assuming that the velocity in each part of the pipe is uniform across the entire cross-section of the pipe, we see that the distance the water travels during a time interval `dt is v1 `dt in the larger pipe, and v2 `dt in the smaller, where v1 and v2 are implicitly assumed the the velocities in these respective parts of the pipe.
• The volume of water flowing in each pipe during this time interval is the volume of the water cylinder whose 'base' area is the cross-sectional area of the pipe and whose 'height' is the distance traveled by the water, as indicated in the figure.
• If the radius of the first pipe is r1 and that of the second is r2, then the two volumes are V1 = `pi r1^2 (v1 `dt) and V2 = `pi r2^2 (v2 `dt), as indicated.
• Since the amount of water flowing through one part of the pipe in any time interval is equal to that flowing through the other, these volumes are equal.
• Setting the volumes equal we see that the ratio of velocities is the inverse square of the ratio of the radii.

An equivalent way of saying all this is the 'continuity equation' A1 v1 = A2 v2, expressing the relationship between flow velocity in cross-sectional area.