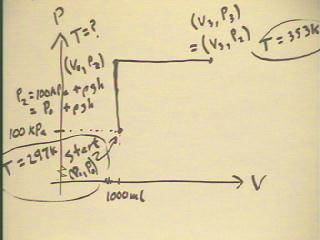
The figure below depicts the pressure vs. volume graph for the bottle engine as water first rises in the tube as the pressure increases at constant volume, then as the gas expands at constant pressure as water is forced out at a constant altitude.

• The system starts at temperature 297 K, and at some unspecified volume V0 and pressure P0 = 100 kPa (approx), and ends up at temperature 353 K.
• As water rises to altitude h, pressure must increase by `rho g h.
• From the pressure ratio between the first and second states we can easily determine the temperature of the second state.
• The volume ratio between the third and second state will be the same as the temperature ratio between the states.

During the first part of the cycle, thermal energy flows into the system as the pressure increases at constant volume; the required thermal energy is found using the molar specific heat at constant volume.

During the second part of cycle, thermal energy flows into the system at constant pressure; the required thermal energy is found using the molar specific heat at constant pressure.

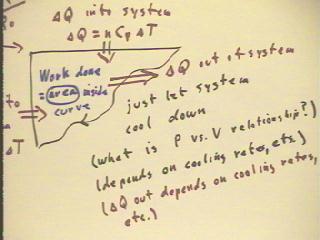
If the system returns to its original state so that another cycle can perform more work, the net work done by the cycle will be the area enclosed by the pressure vs. volume curve.

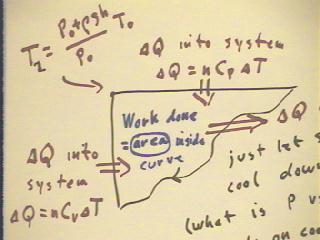
We could just remove the system from the heat source and let it cool down, though this would take some time.

We could if we wanted to run the cycle more quickly release the excess pressure by opening a valve.

We could even find a way to let the gas expand slowly while keeping it at a constant temperature until it reached its original pressure, then let it cool down.

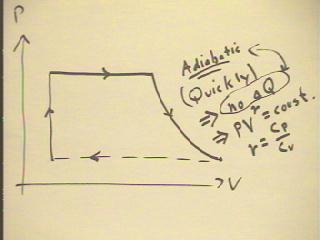
A common way of lowering pressure is to release it by opening a valve.

• This typically releases the pressure so quickly that there is no time for any thermal energy exchange during the expansion.
• A process in which there is no thermal energy exchange is called adiabatic.
• In an adiabatic process, P V^`gamma remains constant, where `gamma is the ratio Cp / Cv of specific sheets at constant pressure and constant volume.

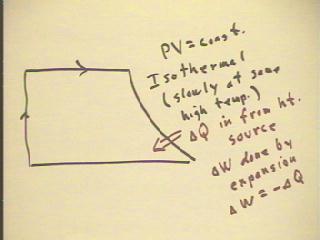
Another way of releasing the pressure, which is generally impractical but is of interest for theoretical reasons, is to allow the gas to expand slowly while adding thermal energy to maintain the constant temperature.

• Such a process is called isothermal.
• In such a process thermal energy is clearly added from the heat source.
• Since the temperature of the gas remains constant, none of the thermal energy added goes into the internal energy of the gas, rather all goes into the work done by the expansion of the gas.

Video File #03

To increase the temperature of a gas, we require thermal energy 1/2 n R `dT to increase the kinetic energy in each of the three independent directions of motion in space, and we require an equal amount for each of the independent directions in which the molecules might gain rotational kinetic energy.

• The three directions of motion in space are generally referred to as the x, y and z directions.
• For diatomic molecules, there are two independent directions perpendicular to the axis of the molecule about which the molecule can rotate with significant kinetic energy.

The total energy the goes into the molecules is called the internal energy of a system.

• We call the energy that goes into motion in the x, y and z directions the translational kinetic energy of the system.
• For a predominantly diatomic gas such as air, which consists of over 90% Nitrogen and Oxygen (both diatomic), there are two more degrees of freedom, the rotational degrees of freedom.
• Thus for a diatomic gas at temperature T we have internal thermal energy 5/2 n R T.

If we wish to expand a gas at constant pressure we must add another 2/2 n R `dT.

• Thus for a diatomic gas to change temperature by `dT requires thermal energy 7/2 n R `dT.
• We thus say that constant-volume specific heat of a diatomic gas is 5/2 R, and the constant-pressure specific heat is 7/2 R.

The figure below summarizes where thermal energy goes into a system consisting of a fixed number of particles of a diatomic or a monatomic gas at constant pressure or constant volume.

• The first term, 3/2 n R `dT, is required to change the translational kinetic energy of the gas particles, which happens for any gas.
• The second term, 2/2 n R `dT, is required to increase the rotational kinetic energy if the gas is diatomic.
• The third term, 2/2 n R `dT, is required to perform the work of expansion if the thermal energy is added at constant pressure.

In an adiabatic process, P V^`gamma remains constant.

• For an ideal diatomic gas, Cv = 5/2 R and Cp = 7/2 R so `gamma = 1.4.
• For an ideal monatomic gas, Cv = 3/2 R and Cp = 5/2 R so `gamma = 1.67.

The figure below once again shows the complete cycle for the bottle engine, with an adiabatic expansion.

• The pressure P3 and volume V3 at the beginning of the adiabatic process are known in terms of the initial pressure P0 and the height h to which water is raised.
• From the values of P3 and V3 we can easily find the value of the constant:  P V^`gamma = const.
• It will then follow that P = const. / V^`gamma, and we can accurately plot the curve corresponding to the adiabatic expansion.
• The work done by the gas during the adiabatic expansion is the area beneath the curve representing the adiabatic process; from this curve the area and hence the work can be estimated.
• Students with a calculus background will understand that the indicated integral is the accurate expression for the work. The integral is easily evaluated.